Uncertainty Quantification Group
- English
- Türkçe
Welcome to the Uncertainty Quantification Group, in the Institute of Applied Mathematics at METU.
Uncertainty quantification (UQ) is a modern inter-disciplinary science that cuts across traditional research groups and combines statistics, numerical analysis and computational applied mathematics. When we attempt to simulate complex real-world phenomena, e.g., fluid dynamics, climate science, chemically reacting systems, oil field research, the price of stock pricing, using mathematical and computer models, there is almost always uncertainty in our predictions. The objective is usually that of propagating quantitative information on the data through a computation to the solution. Our research focuses on advancing fundamental computational methodology for UQ in complex physical systems.
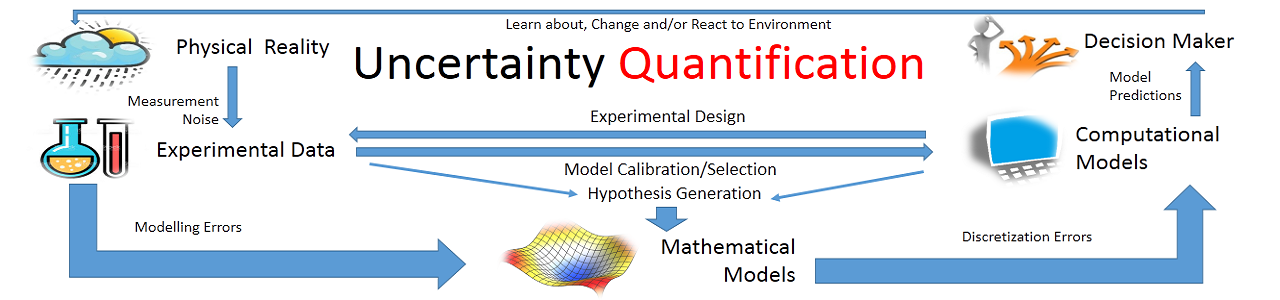
The aim of this research group is to answer the following core questions?
- How to quantify confidence in computational predictions?
- How to build or refine models of complex physical processes from indirect and limited observations?
- What information is needed to drive inference, design, and control?
- How to calibrate and validate our computational models?
In classical setting we solve partial differential equations (PDEs) for a given sets of input data such as material properties, domain geometries, boundary and intial conditions, forcing terms. However, in real-life applications, it is not possible to obtain all this information due to the limited information. If the inputs to the systems under consideration are uncertain, we require mathematical techniques that propagate this uncertainty to the output quantities of interest and methods for computing probabilities of events rather than specific solutions. Our current research includes investigating the numerical solution of partial differential equations (PDEs) with random input data, especially convection dominated problems, for a range of linear and non-linear flow problems.
Team Members:
- Pelin Çiloğlu
- Mustafa Kütük
- Sıtkı Can Toraman
- M. Alp Üreten
- Hamdullah Yücel
Projects:
- Numerical Studies for Petrol and Gas Reservoir Problems (TUBITAK 1001: 119F022, August 01 2019 - August 01 2021)
- Numerical Studies of Korteweg-de Vries Equation with Random Input Data (Proje No: YÖP-705-2018-2820, May 29 2018 - May 29 2019)
Prospective Students:
If you are interested in uncertainty quantification or for any other comments or questions, please visit the webpage for more information and feel free to contact me at yucelh[at]metu.edu.tr. We are always looking for motivated and talented research members.
